Riddler Express: April 29, 2022

David Ding

April 29, 2022

Terminating Fractions Assemble

Explanation:

Pick a terminating decimal number, any terminating decimal number, say 0.43274369. What is its equivalent form in fractions? Well, the easiest way is to divide it by the power of 10 equal to its precision! The precision of a decimal number, as you'll see throughout this solution, is the key in finding the answer. It is simply the number of decimal places (digis after the decimal point) of a number. If it's repeating or irrational, then we would need infinite precision to represent the number. Thankfully, we are only dealing with terminating decimal places, so finite precision suffices. In the example given above, we have 8 decimal places in the number, so precision of 8 is enough and we simply represent the numer as $43274369/{10}^8$. Très facile!

Of course, it is entirely possible that the fraction can be reduced further, but we are only interested in the value of the fraction. It is a good thing too as we would want to prevent double-counting. Therefore, Keeping everything simple, we work with fractions whose denominators that are powers of 10, reduced or not. In this case, the precision of the fraction would be loosely defined here as $N$.

Now let's roll up our sleeves.

Precision is Everything

Let us consider the denominator of 10. This means we are looking at ALL fractions whose numerator is 1, whose decimal value has a finite precision, and whose denominator is no greater than 10. This can only be the following: 1, 1/2, 1/5, and 1/10. What do those fractions have in common? They are factors of 10. In fact, in keeping up with our simplicity setup and not reducing any fractions, we would have: 10/10, 5/10, 2/10, 1/10. Now let's increase the precision by 1 to work with denominators of 100. In addition to 100/100, 50/100, 20/100, and 10/100, which are equivalent to the fractions covered in the 10-case, we would also have 1/100, 2/100, 4/100, 5/100, and 25/100. Do you see the pattern yet?

In fact, all of the fractions whose denominator is of the form ${10}^N$ for some positive integer $N$ that can be written with terminating decimals and reduced to a fraction whose numerator is 1 MUST have the numerator as a factor of ${10}^N$. The number ${10}^N$ has the prime factorization of $2^N{5}^N$. The sum of all factors would therefore be:

$$\begin{array}{rl}& \text{Sum of all factors of }{10}^N\\ & =(2^0+2^1+\cdots +2^N)(5^0+5^1+\cdots +5^N)\\ & =\left[\frac{(2^{N+1}-1)}{2-1}\right]\left[\frac{(5^{N+1}-1)}{5-1}\right]\\ & =\frac{(2^{N+1}-1)(5^{N+1}-1)}{4}\end{array}$$

Where we made use of the geometric series formula above. The rationale behind the first line of equation is that when expanded, it is the sum of all possible factors of ${10}^N=2^N{5}^N$ whereby each term is taking some powers of 2 from 0 to N and then taking some powers of 5 from 0 to N, combining it, and get a number that is a unique factor of ${10}^N$.

Therefore, for any given precision $N$, the sum we are looking for is:

$$\begin{array}{r}\frac{(2^{N+1}-1)(5^{N+1}-1)}{4\times {10}^N}\end{array}$$

To Infinity and Beyond

However, even a terminating decimal can go up to any arbitrary precision, therefore, we must let $N$ go to infinity here. The answer to the puzzle is simply the evaluation of the following limit:

$$\begin{array}{r}\boxed{{\displaystyle \underset{N\to \mathrm{\infty }}{lim}\frac{(2^{N+1}-1)(5^{N+1}-1)}{4\times {10}^N}}}\end{array}$$

Let's evaluate:

$$\begin{array}{rl}& \underset{N\to \mathrm{\infty }}{lim}\frac{(2^{N+1}-1)(5^{N+1}-1)}{4\times {10}^N}\\ & \\ & =\underset{N\to \mathrm{\infty }}{lim}\frac{2^{N+1}{5}^{N+1}-2^{N+1}-5^{N+1}+1}{4\times {10}^N}\\ & \\ & =\underset{N\to \mathrm{\infty }}{lim}\frac{{10}^{N+1}-(2^{N+1}+5^{N+1})+1}{4\times {10}^N}\\ & \\ & =\frac{{10}^{N+1}}{4\times {10}^N}-\underset{N\to \mathrm{\infty }}{lim}\frac{(2^{N+1}+5^{N+1})-1}{4\times {10}^N}\\ & \\ & =\frac{5}{2}-\underset{N\to \mathrm{\infty }}{lim}\frac{(2^{N+1}+5^{N+1})-1}{4\times {10}^N}\end{array}$$

The second term of the expression above is a limit that can be evaluated via bounding:

$$\begin{array}{rl}& \underset{N\to \mathrm{\infty }}{lim}\frac{(2^{N+1}+5^{N+1})-1}{4\times {10}^N}\\ & \\ & \le \underset{N\to \mathrm{\infty }}{lim}\frac{(2^{N+1}+5^{N+1})}{{10}^N}\\ & \\ & \le \underset{N\to \mathrm{\infty }}{lim}\frac{(5^{N+1}+5^{N+1})}{{10}^N}\\ & \\ & =\underset{N\to \mathrm{\infty }}{lim}\frac{2\times 5^{N+1}}{{10}^N}\\ & \\ & =\underset{N\to \mathrm{\infty }}{lim}\frac{2\times 5\times 5^N}{{10}^N}\\ & \\ & =\underset{N\to \mathrm{\infty }}{lim}\frac{10\times 5^N}{{10}^N}\\ & \\ & =10\times \underset{N\to \mathrm{\infty }}{lim}{\left(\frac{1}{2}\right)}^N\\ & \\ & =0\end{array}$$

Putting everything together:

$$\begin{array}{rl}& \underset{N\to \mathrm{\infty }}{lim}\frac{(2^{N+1}-1)(5^{N+1}-1)}{4\times {10}^N}\\ & \\ & =\frac{5}{2}-\underset{N\to \mathrm{\infty }}{lim}\frac{(2^{N+1}+5^{N+1})-1}{4\times {10}^N}\\ & \\ & =\frac{5}{2}-0\\ & \\ & =\boxed{{\displaystyle \frac{5}{2}}}=2.5\end{array}$$

The graph below shows how the sum converges to 2.5 as precision increases towards infinity:

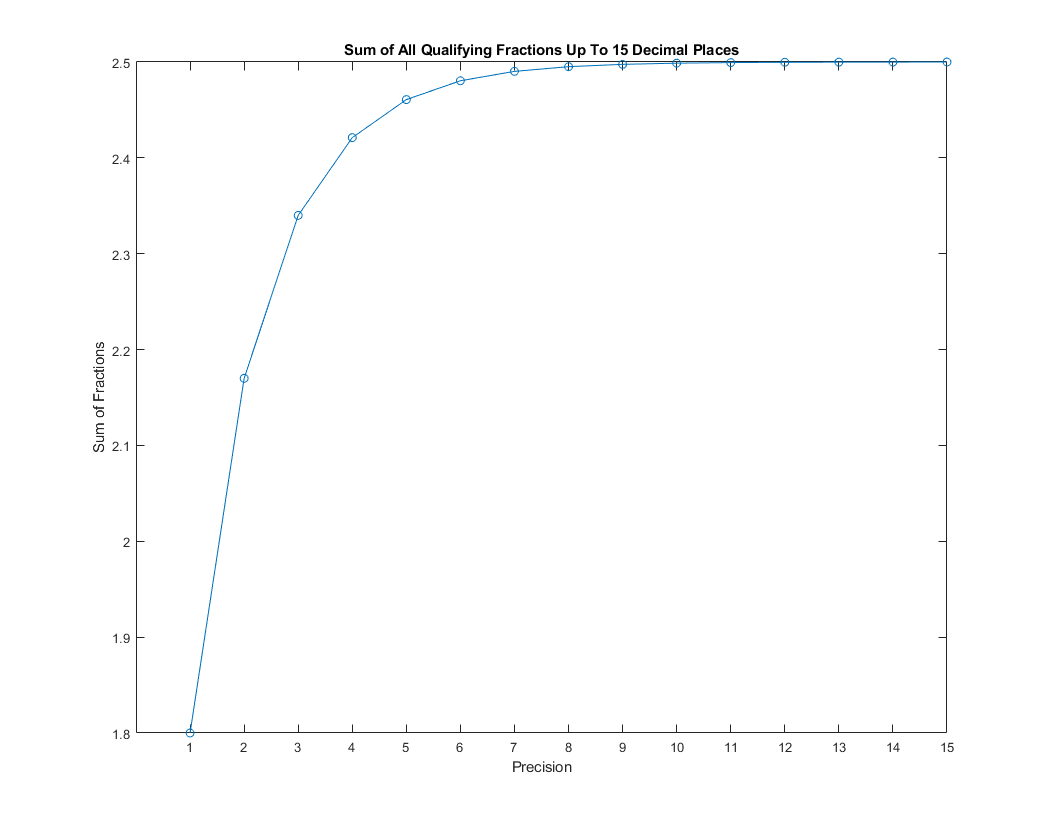
The code is written in MATLAB, and shows the build up of those fractions:

function sumOfFrac = getSum(N)
    % Retrieve the sum of all terminating decimal fractions with precision
    % N
    sumOfFrac = 0;

    for i = 0:N
        for j = 0:N
            denom = 2^i * 5^j;
            sumOfFrac = sumOfFrac + 1/denom;
        end
    end
end

Of course, if 1 is excluded, then the sum still adds up to 1.5, curiously a value that is still greater than 1.